There are multiple ways to calculate A_N. I prefer the cross-ratio method as it takes relative luminosity as well as detector acceptance out of the picture. However, given some of the odd behavior we're seeing it is probably a good idea to examine the asymmetries using another procedure. This could provide a nice sanity check of the methodology, as well as perhaps illuminate some methodology-dependent systematics.

For this study, I have chose to examine the traditional way to calculate A_N. Namely, I look at

A_N = (1/P_beam)×(N^↑-N^↓)/(N^↑+N^↓).

All this requires is to separate the yields into spin states as a function of φ_γγ (where φ_γγ = π/2 - φ_S). The sum over the difference should reveal, in this case, a cos(φ_γγ)-dependence. By leaving the histograms in terms of φ_γγ it also proves a check on my calculation of φ_S.

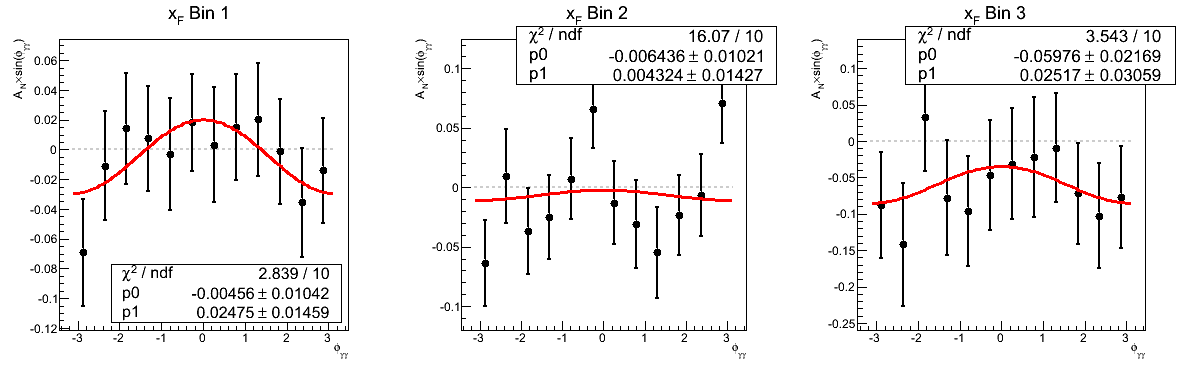
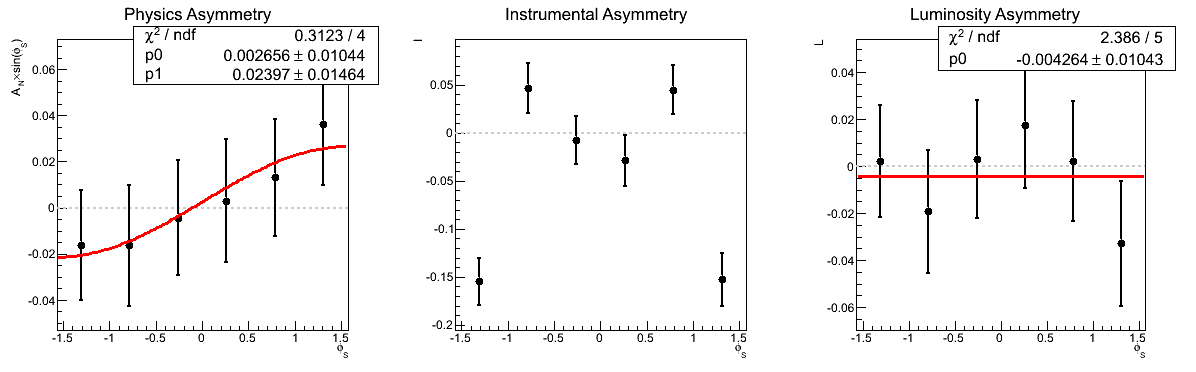
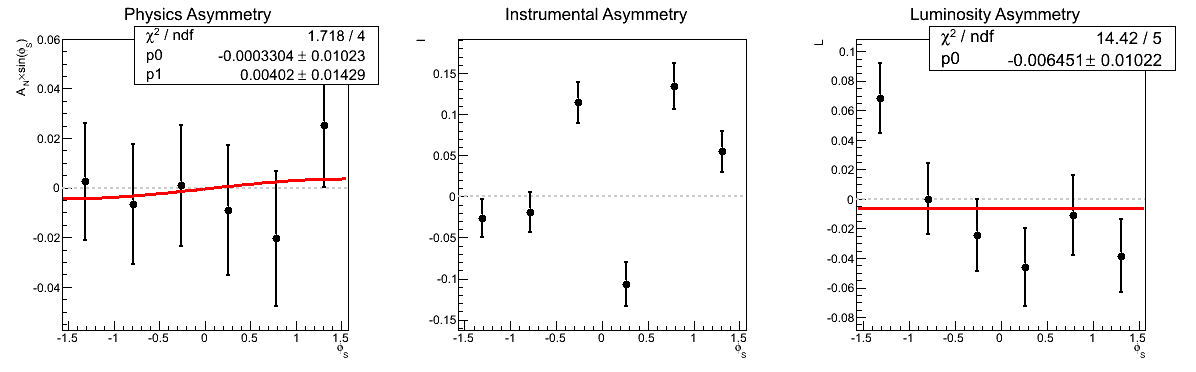
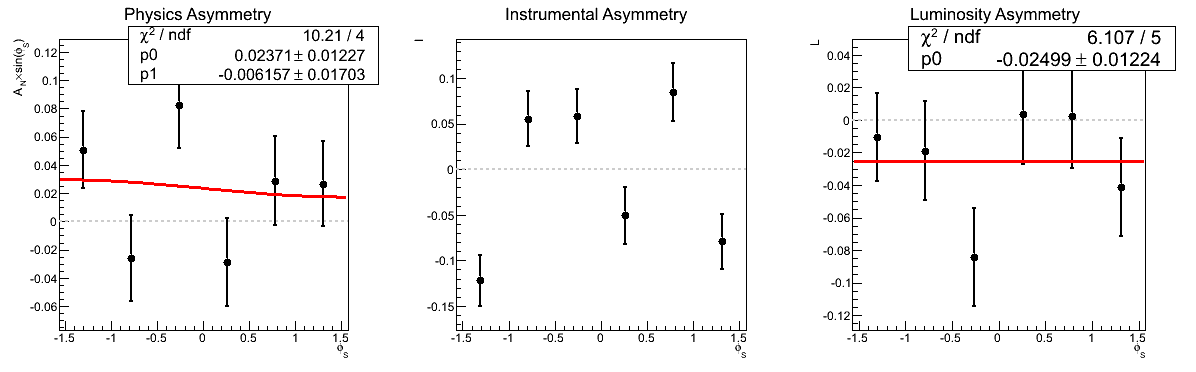
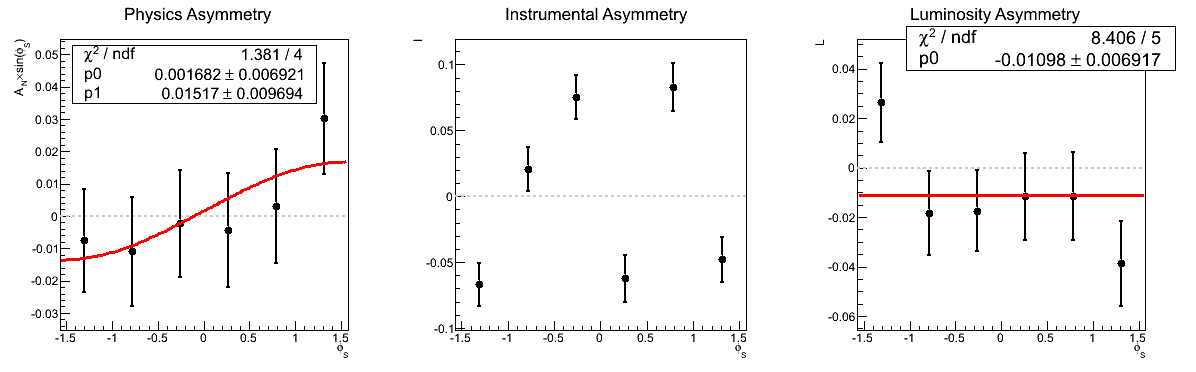
Figure 1: A_N vs. x_F (x_F > 0)

Traditional Method
Cross-ratio Method
xF Bin 1
xF Bin 2
xF Bin 3

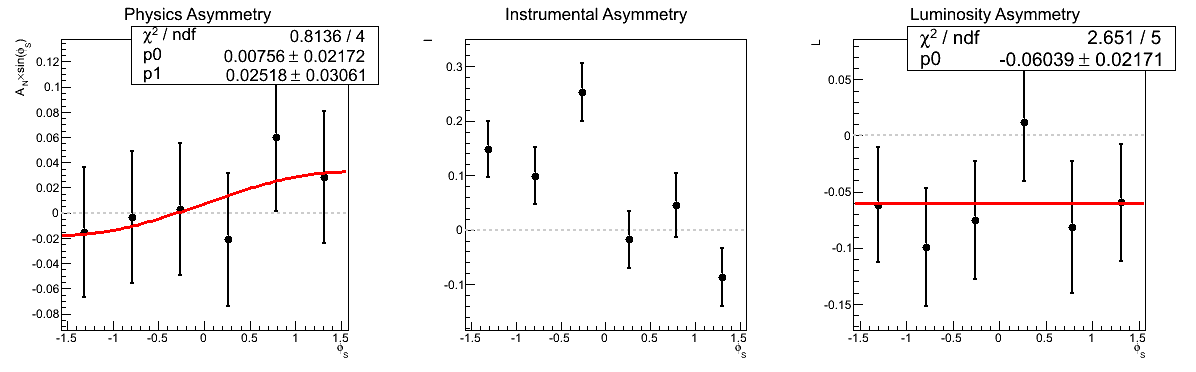In Fig. 1, I show a comparison of the traditional method to the cross-ratio method for the asymmetries as a function of x_F for x_F > 0. One can see that the methods provide completely consistent answers. Additionally, one sees that the p₀'s from the traditional method are equivalent to the luminosity asymmetries from the cross-ratio method. This is indeed as it should be and provides another vote of confidence for our methodology.

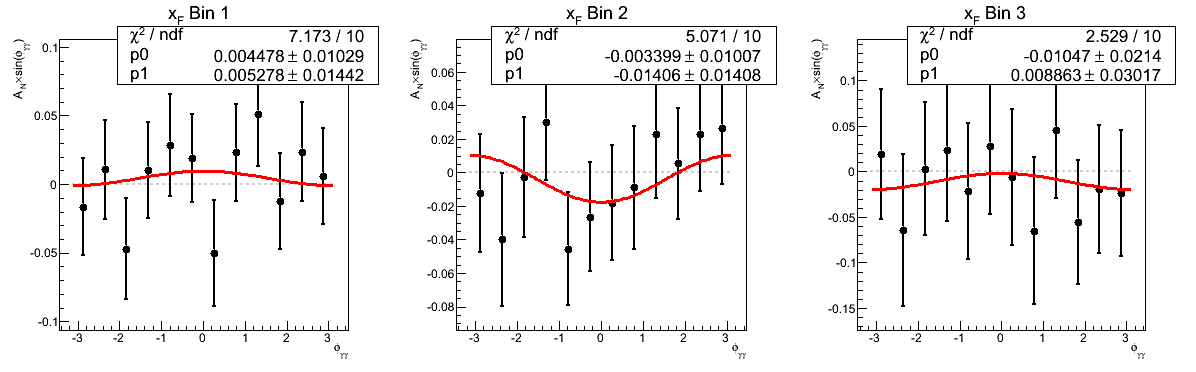
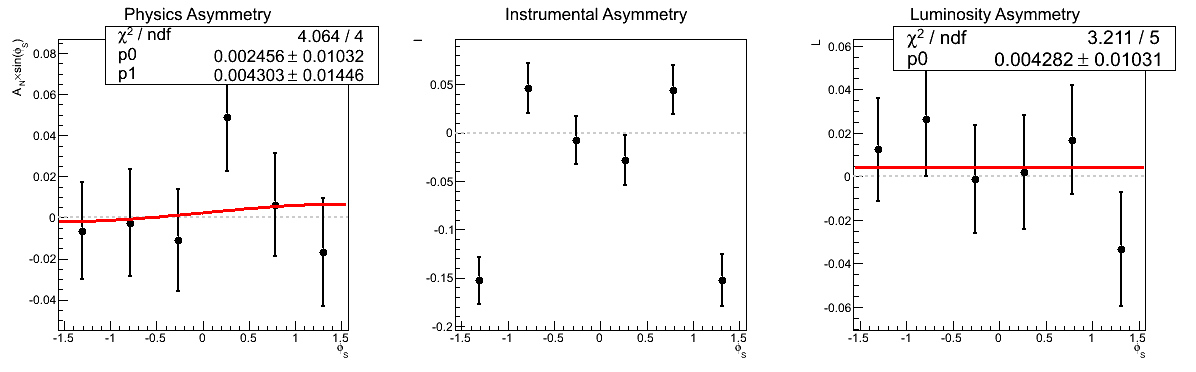
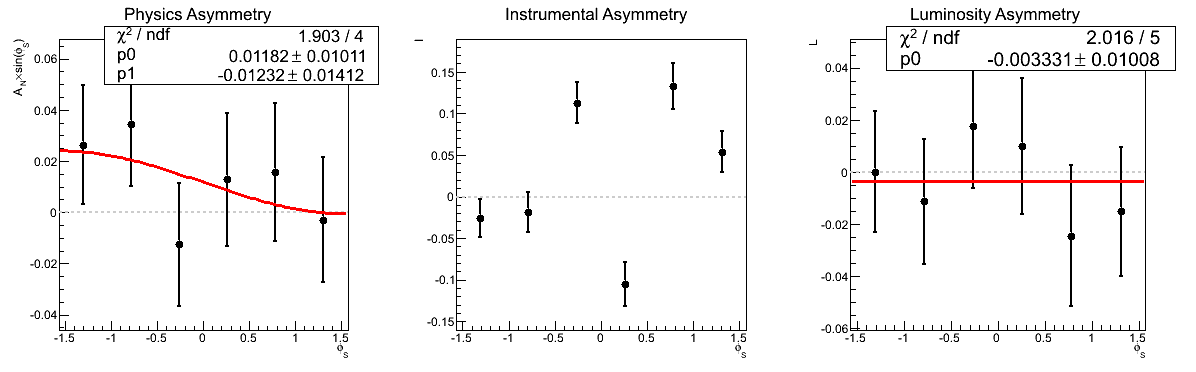
Figure 2: A_N vs. x_F (x_F < 0)

Traditional Method
Cross-ratio Method
xF Bin 1
xF Bin 2
xF Bin 3

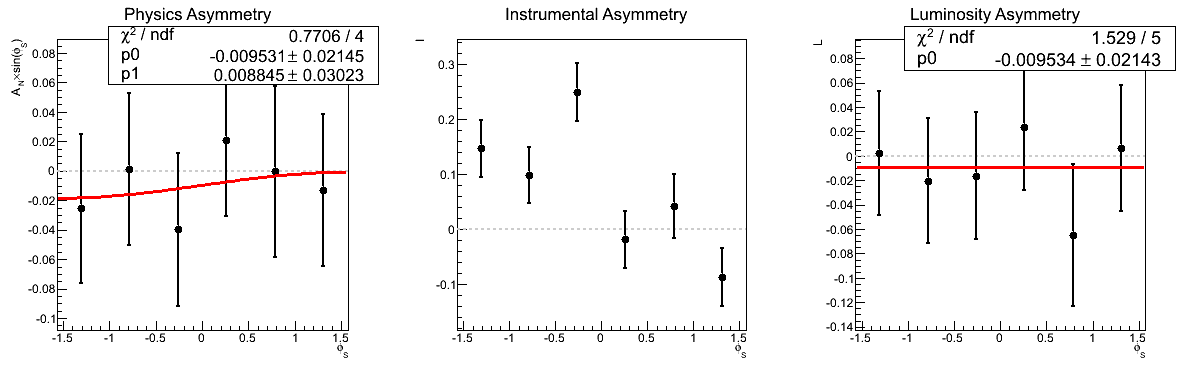In Fig. 2, I show a comparison of the traditional method to the cross-ratio method for the asymmetries as a function of x_F for x_F < 0. Again the methods provide completely consistent answers for both the physics and luminosity asymmetries.

At this point, I will revert back to calculating the asymmetries in terms of φ_S. As I stated earlier, they are simply offset from each other by π/2. Having established that we have calculated φ_S robustly, it should be less confusing, now, to have consistent notation.

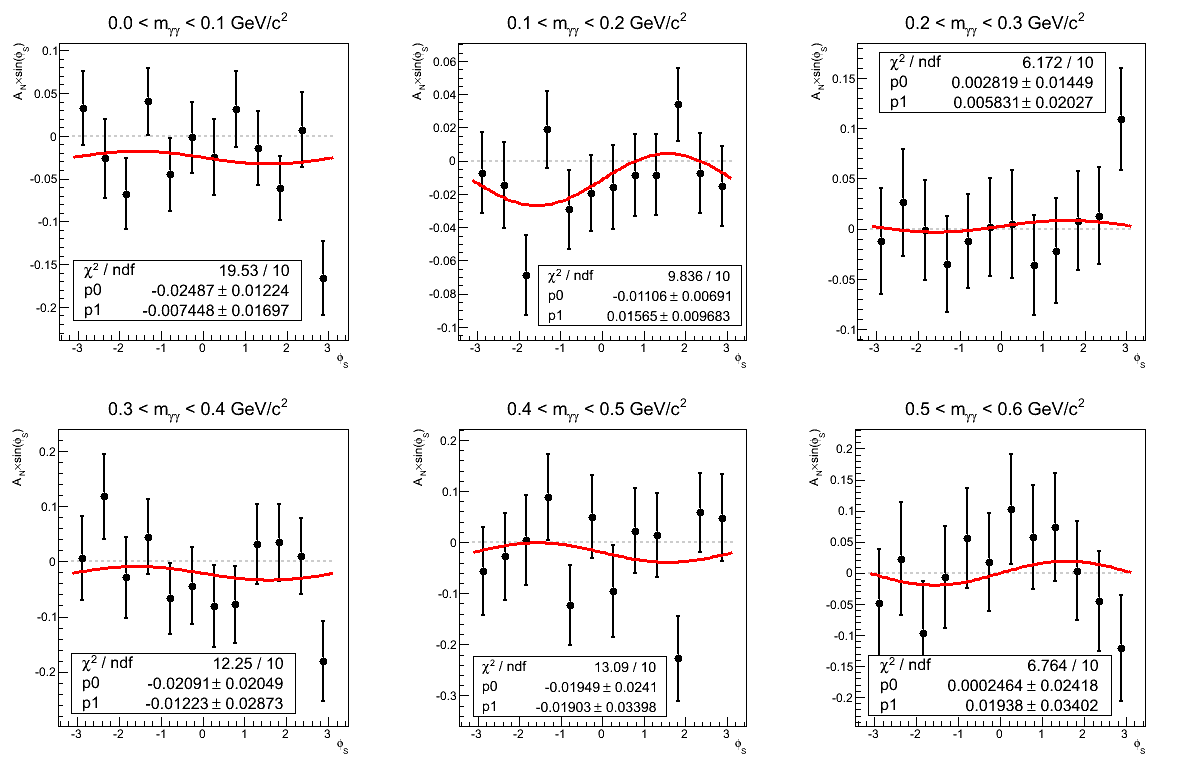
Figure 3: A_N vs. m_γγ (x_F > 0)

Traditional Method
Cross-ratio Method
0.0 < mγγ < 0.1 GeV/c2
0.1 < mγγ < 0.2 GeV/c2
0.2 < mγγ < 0.3 GeV/c2
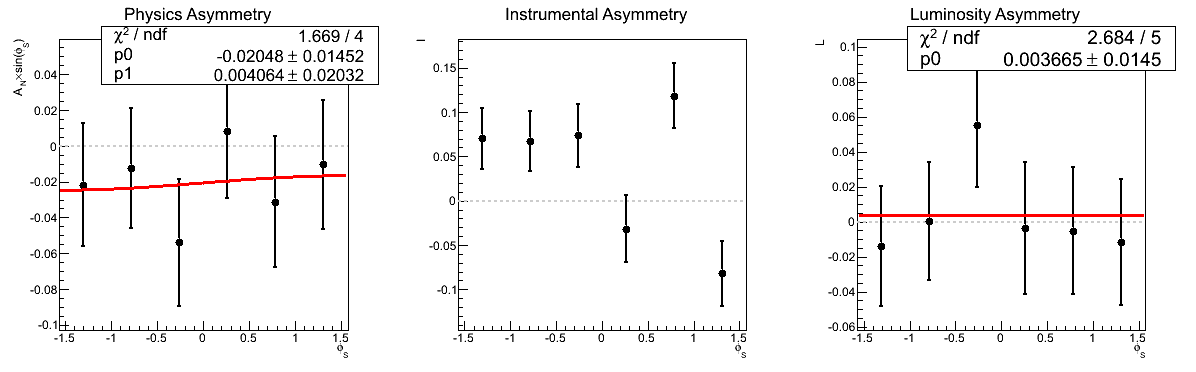0.3 < mγγ < 0.4 GeV/c2
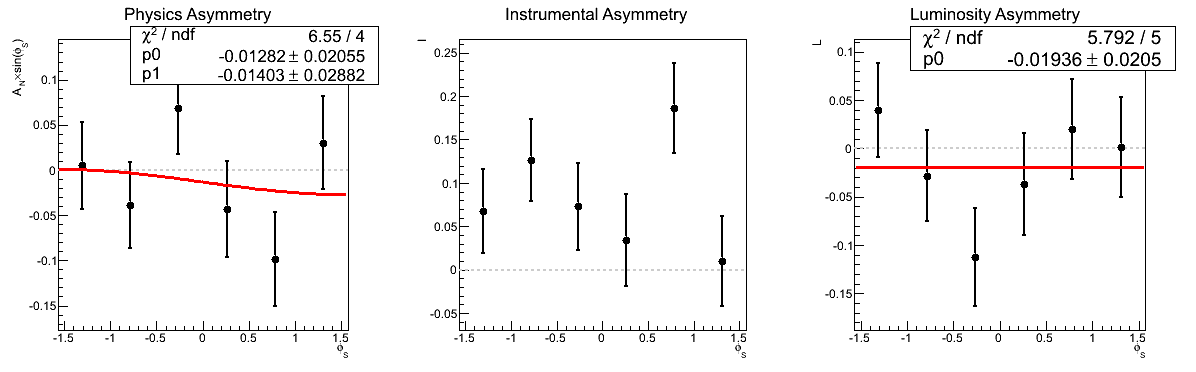0.4 < mγγ < 0.5 GeV/c2
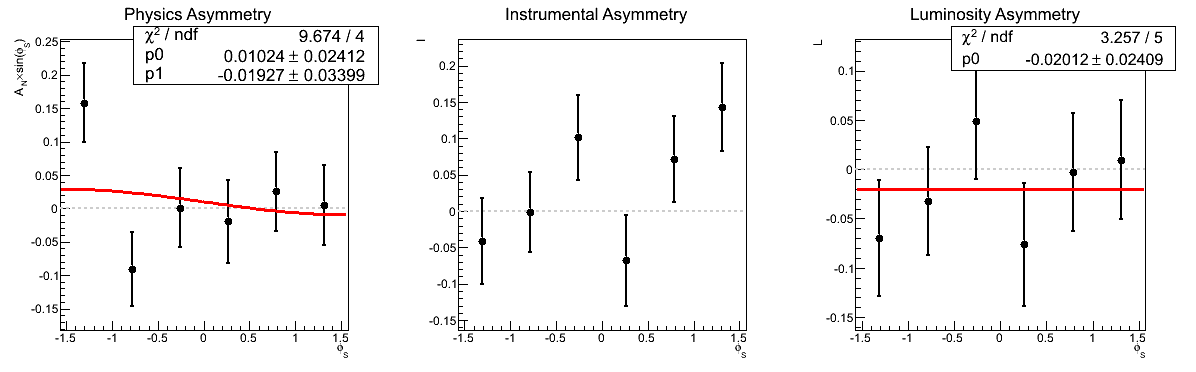0.5 < mγγ < 0.6 GeV/c2

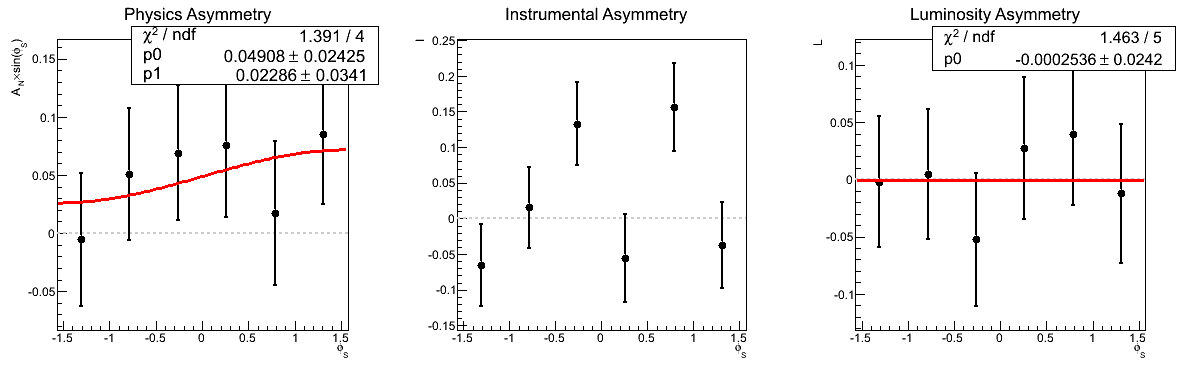Figure 3 shows the asymmetries as a function of invariant mass for x_F > 0. Again, the methods are consistent. Note that in the mass bin corresponding to the π⁰ the methods are entirely equivalent within statistics and differ numerically by only 3.1% (0.035σ).

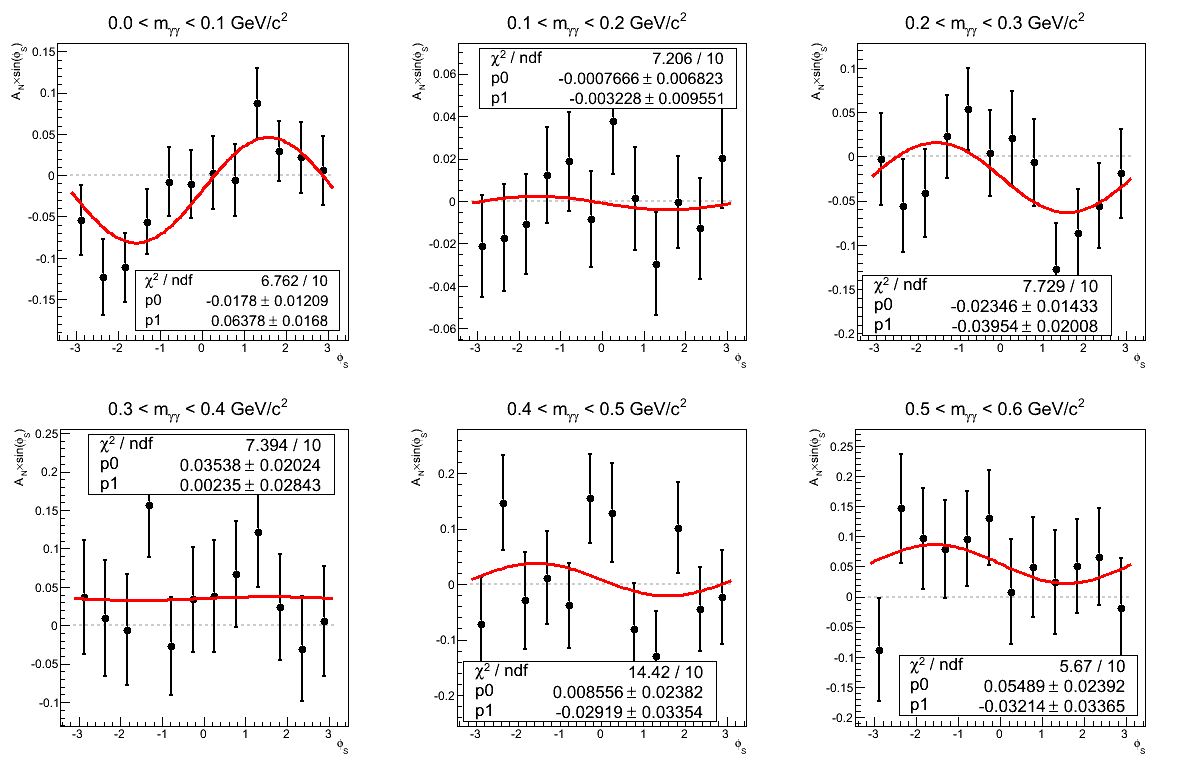
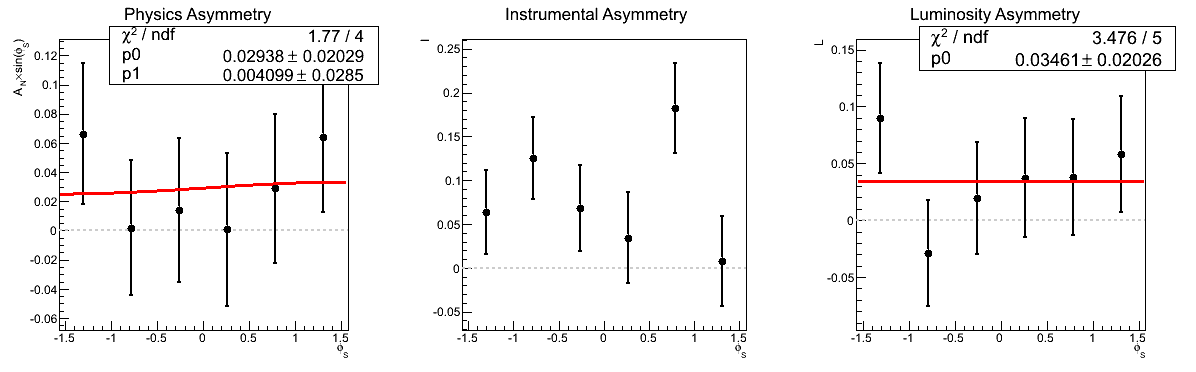
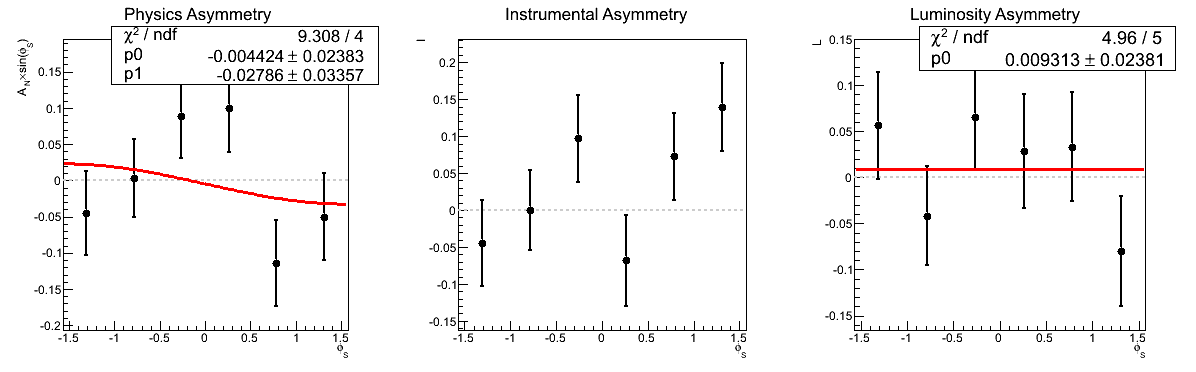
Figure 4: A_N vs. m_γγ (x_F < 0)

Traditional Method
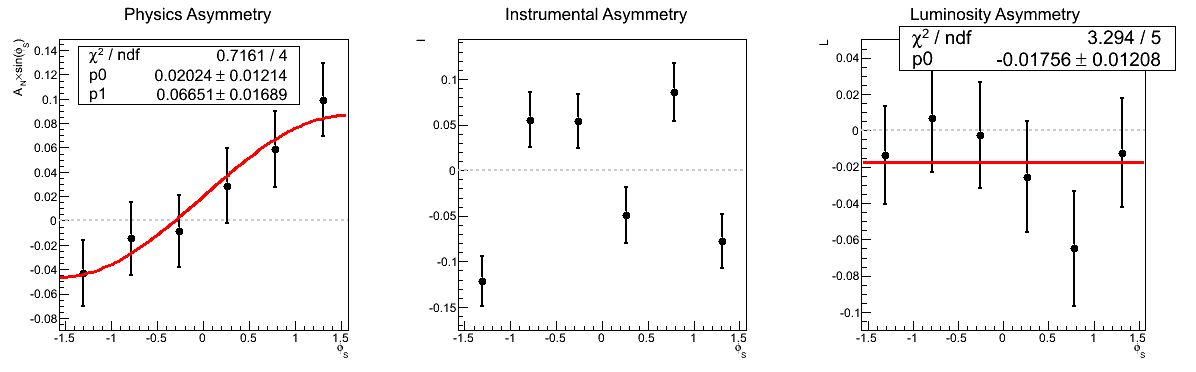
Cross-ratio Method
0.0 < mγγ < 0.1 GeV/c2
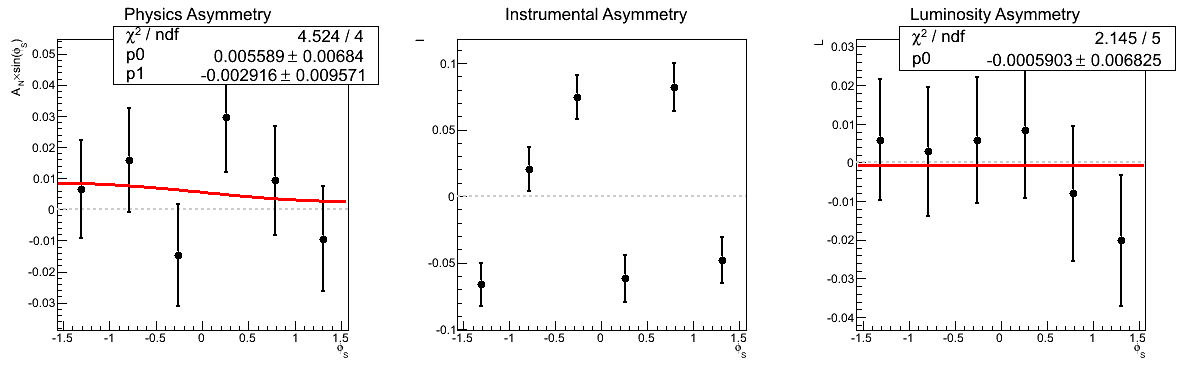
0.1 < mγγ < 0.2 GeV/c2
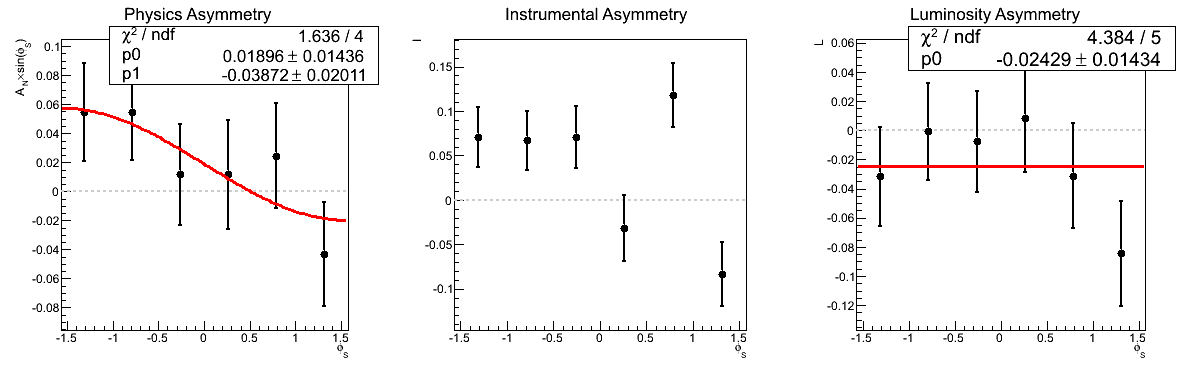
0.2 < mγγ < 0.3 GeV/c2
0.3 < mγγ < 0.4 GeV/c2
0.4 < mγγ < 0.5 GeV/c2
0.5 < mγγ < 0.6 GeV/c2

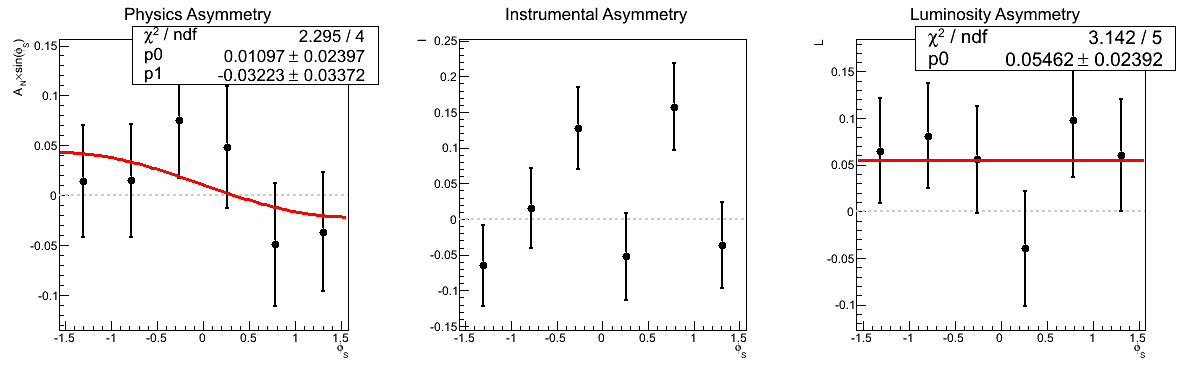In Figure 4 I show the asymmetry as a function of invariant mass for x_F < 0. Again, the methods yield consistent results, even for the lowest mass bin. Thus, I cannot conclude at this point that the odd behavior in that bin is due to the methodology. It still may be the result of something stupid on my part, however, I can't conclude that based on this study.